The thermal radiation of the black body
PDF
Why does the colour of a metal change when it is heated? How can the temperature of a light source such as a tungsten filament lamp or the surface of the sun or stars be determined? What is the temperature of the Universe? What is the principle of a thermographic camera? The answers to these questions are based on the analysis of the radiation of a black body, i.e. a body that absorbs all the light it receives. Planck’s theory explaining this radiation was the first step towards the development of quantum mechanics.
1. The different types of radiation
There are several types of radiation, i.e. the emission of waves. We can quote:
- The sound waves emitted by a person who speaks, a musical instrument or a loudspeaker, the vibrating quantity that propagates with the wave being then the pressure.
- Seismic waves are elastic waves that can pass through a medium by modifying it according to the intensity of the earthquake.
- Electromagnetic waves which, as their name suggests, are characterized by an electric field E and a magnetic field B which vibrate with a certain frequency. These fields are perpendicular to each other and to the direction of wave propagation.
In the article the colour of the sky, electromagnetic waves have been described in detail. A particular electromagnetic wave propagating in a given environment is characterized by its frequency ν, the amplitude and direction of its electric field, its propagation velocity (or celerity) V in the environment considered. In vacuum this speed is c ≃ 300 000 km s-1. The wavelength is λ = V/ν : it is the distance travelled by the wave at speed V during a period T = 1/ν. The frequency is expressed in hertz (Hz), i.e. in periods/second noted more simply s-1. The intensity of an electromagnetic wave is proportional to the square of the amplitude of its electric field. It represents the flow of energy carried by the wave. The orientation of the electric field, when fixed, is its polarization.
The different electromagnetic waves are classified according to their frequency ν, or equivalently according to their wavelength in vacuum λ = c/ν. The wavelength range is very wide: from 103 m for large radio waves to 10-12 m for γ rays. The spectrum visible to our eye is limited to wavelengths between 0.4 µm for violet and 0.8 µm for red (1 µm=1/1000 mm).
Electromagnetic waves are created by rapid oscillations of electrical charges. Radio waves (λ ~ 100 m), television waves (λ ~ 10 m), those used by mobile phones and WIFI (Wireless Fidelity, λ ~ 0.10 m), are emitted by antennas traversed by exciting electric currents. Shorter lengths, such as X-rays (λ ~ 10-10 m), can be obtained either by accelerating or braking electrons in vacuum at different energies or by being emitted by previously excited atoms. The γ rays result from nuclear reactions (see Radioactivity).
The atoms or molecules of a gas excited by an energy supply emit electromagnetic radiation at specific wavelengths characteristic of the nature of the gas (this is how the chemical composition of the star surface is known). This is called a line spectrum. There is also a so-called thermal radiation, which any body emits merely because of its temperature. This radiation is distributed over a continuous range of wavelengths, known as a continuous spectrum. This is the case of a filament lighting lamp, the Sun or stars and more generally the surface of a body heated to a certain temperature. To analyze this radiation, it is necessary to study the properties of the black body defined in the following section.
2. The internal radiation of the black body
A black body is an ideal object that would absorb all the electromagnetic energy it would receive, without reflecting or transmitting it. Thus illuminated, it would totally absorb light and should appear black, hence its name. However, it only appears black at low temperatures, because it emits light, with a flux strongly increasing with temperature; it is this phenomenon that is described here.
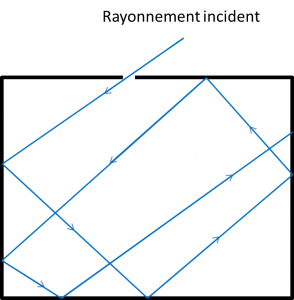
A black body is well represented by a small hole drilled in a cavity heated at an absolute temperature T (e. g. a furnace). The hole must be small enough not to disturb the balance in the cavity (Figure 1). Indeed, if the hole in the wall is small enough, any radiation from the outside that enters the cavity is reflected several times on the walls and has a very low probability of exiting through the hole. The radiation from the hole to the outside can then be experimentally analysed as a function of the frequency ν of the waves emitted and the temperature T of the cavity walls .
In this cavity, empty of any gas to simplify, the atoms composing the walls permanently emit electromagnetic radiation and absorb that emitted by the other atoms of these walls. This emission can be explained qualitatively in a classical way by movements of charged particles. Each atom is formed by the positively charged nucleus and the electronic cloud of negative charge that gravitates around it. It behaves like an electrostatic dipole, i.e. a positive charge associated with a negative charge. Under the effect of temperature, this dipole oscillates randomly and behaves like a microscopic antenna, excited by a micro-current, which radiates energy. This continuous spectrum radiation occupies the entire cavity. Conversely, the electric field of the waves sets these charges in motion and thus gives them energy, which constitutes absorption. A state of equilibrium is quickly reached so that during a unit of time the energy emitted by the atoms is equal to the absorbed energy.
Thus the electromagnetic energy density ρ(T) (energy per unit volume of the cavity) is constant. This energy density comes from all waves with a frequency from 0 to infinity. It can be seen that ρ(T) is proportional to the power 4 of the absolute temperature T, i. e. ρ(T) = αT4. The total energy, and therefore the radiation emitted, increases considerably with temperature. Thus the hole appears very bright when the cavity is at high temperature and completely black at very low temperature.
Each contribution to energy density at the frequency ν, ρν(ν,T), constitutes what is called the spectral energy density. Experience shows that it is the same at every point in the cavity and that it is independent of its geometric shape and volume as well as of the material that constitutes its walls: it is a universal function of ν and T. To measure this function, simply drill a small hole in the cavity, and analyze the radiation that comes out as a function of ν and T.
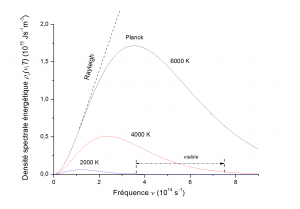
At each temperature it is observed that the function ρν(ν,T) follows a bell curve as a function of frequency (Figure 2) with a pronounced maximum for a frequency value νm = 5.879 ×1010T (s-1) which increases linearly with temperature expressed in degrees K (Kelvin). This explains the change in colour of a radiating body: iron is dark red at 680°C (953 K), light red at 810°C, yellow at 1000°C and white at 1200°C [1].
3. Energy radiated by a black body
The energy density inside a cavity is a quantity which is difficult to measure directly, and instead the energy flow radiated by a small surface hole S is accessed. This flux can be measured by placing a sensor in front of the hole whose heating rate will represent the energy of the absorbed radiation. The flow of energy radiated through a surface is defined as the energy crossing that surface per unit of time and area. If all the radiation escaped perpendicular to the surface S at velocity c (Figure 3a), the content of the volume Sc dt would pass through the surface in a time dt. The corresponding energy would therefore be ρSc dt. By dividing by time and surface, we would obtain an energy flow ρc directly related to the total energy density ρ.
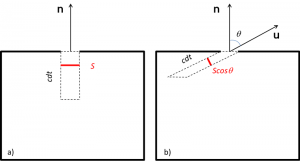
In reality, the outflow through the surface S is affected by a factor ¼ whose origin is geometric. Indeed, inside the cavity, the radiation is distributed in all directions in an isotropic manner by multiple reflections. Thus only a small part of the radiation exits in the vicinity of a direction represented by the unit vector u at an angle θ with the normal n (Figure 3b). More precisely in a very small solid angle[2] dΩ around u, only the proportion dΩ/4π of the radiation can intervene. In addition, as in the previous case, the content of the oblique cylinder in Figure 3b that would pass through the surface in a time dt must be considered. This cylinder has a normal cross-section at the propagation direction S cosθ and a length c dt. This volume is therefore S cosθ cdt. The elementary flow per unit of time and area output is therefore dΦ = ρ = ρ c cosθ dΩ/4π. The sum on all directions of radiation, i. e. on the half-space outside the hole, of cosθ/4π being equal to ¼, we obtain a total energy flux given by Stefan-Boltzmann’s law discovered empirically in 1871:
Φ = ρ c/4 = σT4 with σ = 5.670×10-8 W.m-2.K-4
The contributions to this flux of frequency radiation ν are equal to ρν(ν,T)c/4 and can be measured. It should be noted that the radiation emitted by the black body is completely independent of incident radiation that would penetrate through the hole and be totally absorbed. The theoretical foundations of this radiation were laid in thermodynamics by Stefan’s doctoral student Ludwig Boltzmann in 1884, but were only elucidated by Max Planck in 1901 using quantum theory (see focus).
4. Emission and absorption, Kirchhoff’s law
By definition, a surface is black in a certain frequency domain if any electromagnetic radiation in that frequency domain is absorbed. Nevertheless, this surface re-emits electromagnetic waves by thermal radiation. The flux Φ radiated by a surface S at temperature T is equal to the flux emitted by a small hole of the same surface in a cavity at the same temperature. Indeed, if the hole is closed by the black surface (Figure 3), at thermal equilibrium, the flow from the black surface to the inside of the cavity must be equal but opposite to the average heat flow from the cavity absorbed by the black surface.
Similarly, if a non-black object at temperature T absorbs a fraction a of the incident radiation that falls on it, the radiation flux emitted by the object at the same temperature will be once the flux emitted by a black body at that temperature. The factor a refers to absorptivity. The emissivity, which is the ratio between the emission of radiation from a surface and that of a black body at the same temperature, is denoted by the factor e. Kirchhoff‘s law states that these two factors are equal, i.e. a = e. For a perfect reflector like a mirror, a = 0, therefore e = 0: a perfect reflector does not radiate thermally.
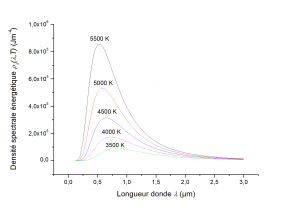
Often it is preferable to express the spectral energy density of the black body as a function of the wavelength λ = c/ν rather than the frequency ν of the waves. This function ρλ(λ,T) is easily obtained from ρν(ν,T) (see focus). The graph of this function, which is represented as a function of λ for various temperatures in Figure 4, looks like a bell curve.
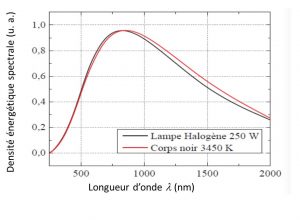
Figure 5 shows the radiation of a halogen lamp that corresponds well to that of a blackbody (e = 1) at 3450 K.
At ordinary temperature the materials radiate in the infrared. The emissivities of materials depend on the wavelength but also on the state of their surface. Typically [3] for a polished aluminium foil e (λ = 3 µm) = 0.09 and e (λ = 10 µm) = 0.04. If we look at the total emissivity e integrated at all wavelengths we have, for example, e = 0.02 for polished gold, e = 0.60 for sand, e = 0.80 for snow, e = 0.96 for liquid water, e = 0.70-0.90 for white paper, e = 0.90 for black paper and e = 0.98 for human skin. Soils and liquid water have emissivities close to 1, which facilitates the determination of ground temperatures by infrared imaging of satellites.
5. Wien’s Law and applications to astrophysics
The ρλ(λ,T) curves shown in Figure 4 present a maximum for λm given by Wien’s law:
λmT = 0.201 hc/kB = 2.896×10-3 m.K
where h is the Planck constant, c is the speed of light in vacuum and kB is Boltzmann’s constant (read Pressure, temperature, heat). This very simple expression is used to estimate surface temperatures.
–Temperature of the solar surface
The solar surface can be considered as a first approximation as a black surface; the density ρλ(λ,T) of the solar radiation is maximum for λm = 0.501 µm. From Wien’s law it is then possible to deduce the temperature of the solar surface TS = 5777 K.
–Heat flux radiated by the Sun to the Earth

By assimilating the solar surface to a black surface, the flux emitted by the Sun is according to Stefan-Boltzmann’s law Φ0= σT4S. The total power P0 emitted by the Sun of radius R0 = 7×105 km is therefore the product of this flux by the surface of the sphere of radius R0, P0 = 4πR02Φ0 At a distance L from the Sun, the same power is distributed over a sphere of radius L, which represents a local flux Φ’ = P0/(4πL2) = Φ0 (R0/L)2. At the average distance from the Earth L = 1.5×108 km, we obtain a flux of Φ’ = 1367 W/m2. Figure 6 shows the actual emission spectrum of the Sun measured outside the atmosphere and which can be compared to its theoretical expression from Planck’s law, proportional to ρλ(λ,T) for T = 5777 K. The few most notable deviations from the theoretical blackbody curve are due to the emission and absorption lines of the elements present on the solar surface, mainly Balmer’s lines [4], of hydrogen.
–Radiation temperature of the Earth’s surface
This subject is covered in the article The Earth’s gaseous envelope.
–Fossil radiation from the Universe
Penzias and Wilson discovered in 1964 the existence of fossil radiation similar to that of a black body with a maximum at λm = 1.06 mm which corresponds to a temperature of 2.73 K. This radiation has a very low anisotropy, i.e. it varies very little according to the orientation of the receiving antennas. This observation provides important support for the big-bang model, which assumes an expanding Universe that cools over time. According to this theory, cosmic radiation has persisted since the primary period when the Universe was composed of electrons and protons at about 4000 K. This plasma of electrons and protons interacted strongly with electromagnetic radiation at all frequencies, so that matter and radiation were in equilibrium. The Universe then cooled down to 3000 K forming a material composed essentially of hydrogen atoms. The interaction with the black radiation was only carried out at the frequencies characteristic of hydrogen. Most of the electromagnetic radiation was therefore decoupled from matter and the Universe cooled by adiabatic expansion to 2.73 K (similar to the expansion of a gas). After the previous decoupling, matter evolved into heavier atoms that organized themselves into galaxies, stars, dust clouds. The electromagnetic radiation from these objects is added to the cosmic radiation of the black body.
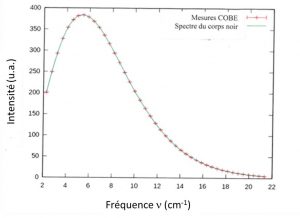
Figure 7 shows the flux spectrum of this cosmic radiation as a function of frequency, measured using the COBE satellite [5] in 1989. The theoretical curve proportional to the energy spectral density ρν(ν,T) given by Planck’s formula (see focus) is perfectly superposed on the experimental points for T = 2.726 K. It should be noted that fossil radiation is present throughout the universe and currently corresponds to a total radiation of about 500 photons/cm3.
6. Application to thermographic cameras
A thermographic camera [6] records the different infrared rays that vary according to the temperature of the emitting body. It thus makes it possible to measure the temperature from the law of blackbody radiation. A removable filter allows the radiation to be integrated into spectral atmospheric transparency windows. The system converts the radiation power into digital or analog signals: these are transcribed into temperature by the computer and transformed into an image. The image thus obtained is called “thermogram”.
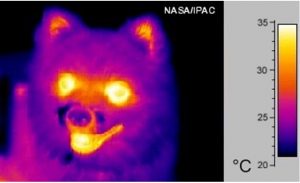
The wavelength of infrared radiation depends on temperature; at room temperature, around 300 K, the maximum radiation given by Wien’s law is around λ ~ 10 µm. The sensor of these cameras measures the received infrared radiation which is the sum of the proportional contributions to the function ρλ(λ,T) in the selected wavelength range in the vicinity of λ = 10 µm. The colour produced by the camera is a false colour, obtained by associating a colour with the intensity received in order to facilitate the direct reading of the temperature: each colour in the image has a corresponding temperature as in the thermal image on the cover photo of this article or the thermal image of a dog in Figure 8.
A thermal camera can be used in many situations: by firefighters to search for earthquake victims and their location in hard-to-reach places or to detect emerging or smoldering fires, by companies to detect weak points in building insulation, in airports to detect people with suspected fever, for the study of nocturnal species such as bats without disturbing them with a visible light source.
References and notes
Cover image. Thermal image of an English subway mouth. [Source: branestawm2002 via Visual Hunt / CC BY]
[1] http://www.thethermograpiclibrary.org/index.php?title=Table_emissivated_in_thermography
[2] In the same way that an angle represents the length of an arc of a circle of unit radius, a solid angle represents a portion of a surface on a sphere of unit radius. For an observer at a point O, the solid angle at which an object is seen is the projection of the apparent surface of the object onto a sphere of a radius unit centred at O. For example, inside a closed cavity, the solid angle at which the cavity is seen is none other than the total surface area of a sphere of unit radius, i. e. 4π
[3] http://www.thethermograpiclibrary.org/index.php?title=Table_emissivated_in_thermography
[4] Elie Belorizky Introduction to Quantum Mechanics, Dunod, Paris, 2003.
[5] https://en.wikipedia.org/wiki/Cosmic microwave background
[6] Not to be confused with the so-called infrared cameras used for simple night vision, operating at shorter wavelengths (1 µm), which does not allow thermal measurements.
The Encyclopedia of the Environment by the Association des Encyclopédies de l'Environnement et de l'Énergie (www.a3e.fr), contractually linked to the University of Grenoble Alpes and Grenoble INP, and sponsored by the French Academy of Sciences.
To cite this article: BELORIZKY Elie, PIQUE Jean-Paul (January 5, 2025), The thermal radiation of the black body, Encyclopedia of the Environment, Accessed January 18, 2025 [online ISSN 2555-0950] url : https://www.encyclopedie-environnement.org/en/physics/thermal-radiation-of-black-body-2/.
The articles in the Encyclopedia of the Environment are made available under the terms of the Creative Commons BY-NC-SA license, which authorizes reproduction subject to: citing the source, not making commercial use of them, sharing identical initial conditions, reproducing at each reuse or distribution the mention of this Creative Commons BY-NC-SA license.







