黑体的热辐射

为什么金属受热时会变色?如何确定白炽灯等光源或太阳等恒星表面的温度?宇宙的温度有多高?热成像相机的原理是什么?这些问题的答案都基于对黑体辐射的分析。黑体指的是一类物体,其能够吸收自身接收到的所有光,解释黑体辐射的普朗克理论是量子力学发展的第一步。
1. 不同类型的辐射
辐射(radiation),即波的发射有几种类型,主要包括:
- 声波(sound wave),由说话的人、乐器或扬声器发出,随着波传播的振动量是压强。
- 地震波(seismic wave)是弹性波,可以通过改变介质的方式穿过介质,改变的程度取决于地震的强度。
- 电磁波(electromagnetic wave),顾名思义,是以一定频率振动的电场E和磁场B为特征的波。这些场相互垂直,且与波的传播方向垂直。
《天空的颜色》一文对电磁波进行了详细的描述。在给定环境中传播的特定电磁波的特征包括其频率ν、其电场的振幅和方向、其在环境中的传播速度(即波速)V。在真空中,该速度c≃ 300000 km s-1。波长λ = V/ν,指的是在T = 1/ν周期内,速度为V的波传播的距离。频率的单位是赫兹(Hz),即每秒(一般写作s-1)的周期数。电磁波的强度与其电场振幅的平方成正比,代表了波所携带的能量流。如电场的方向固定,则称这种固定电场为波的极化。
不同的电磁波可根据其频率ν,或等价地根据其在真空中的波长λ = c/ν进行分类。不同的电磁波波长相差巨大:大型无线电波的波长可达103 m,而γ射线的波长则仅有10-12 m。可见光光谱仅包含波长0.4 µm的紫色和0.8 µm的红色之间的一小部分(1 µm = 1/1000 mm)。
电磁波是由电荷的快速振荡产生的。无线电波(λ ~ 100m)、电视波(λ ~ 10m)、手机和WIFI(wireless fidelity,无线局域网)使用的无线电波(λ ~ 0.10m)是由激发电流穿过天线发射的。而波长较短的波,如X射线(λ ~ 10-10m),则可以通过在真空中以不同的能量加速或制动电子产生,或者由先前激发的原子发射。γ射线是由核反应产生的(见《放射性》)。
气体的原子或分子在受到能量源激发后,会发出特定波长的电磁辐射。这是气体的性质之一,借助该性质,能够获知恒星表面的光谱(line spectrum),进而分析其化学成分。此外,任何物体都会因为具有温度发出电磁辐射,称为热辐射(thermal radiation)。这种辐射分布在连续的波长范围内,称为连续光谱(continuous spectrum)。白炽灯、太阳或星星,以及一切加热到一定温度的物体表面都会发生上述现象。为了分析这种辐射,有必要了解黑体的特性。
2. 黑体的内部辐射
黑体(black body)是一种理想物体,它会吸收自身接收到的全部电磁能量,而不会发生反射或传输。因此,黑体在受到光照时将完全吸收光线,并呈现出黑色,“黑体”正是由此得名。然而,其只在低温下呈现黑色,在高温下则会发光,且随着温度的升高,通量明显增加。
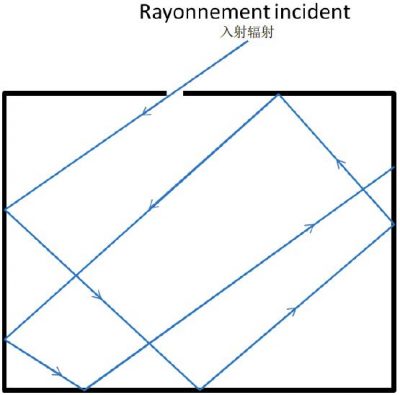
黑体的一个典型代表是处于绝对温度T下的、钻了小孔的空腔,类似于熔炉。小孔必须足够小,以免干扰空腔中的平衡(图1)。事实上,如果壁上的孔足够小,任何从外部进入空腔的辐射都会在壁上发生多次反射,并且通过孔射出的可能性非常低。从孔到外部的辐射可以用关于发射波频率ν和腔壁温度T的函数进行实验分析。
在这个不存在任何气体的简化空腔中,构成腔壁的原子会永久地发出电磁辐射,并吸收壁上的其他原子发出的辐射。这一现象可以用经典的带电粒子运动来定性解释。每个原子都是由带正电荷的原子核和周围的负电荷电子云组成的,行为类似于静电偶极子(dipole),即一对相距很近的相反电荷。在温度的作用下,该偶极子随机振荡,如同被微电流所激发的微型天线一般,向外辐射能量。这种连续光谱辐射占据了整个空腔。与之相对,辐射波的电场可以引发电荷运动,并为其供能,偶极子因此可以向内吸收能量。两种过程很快达到平衡状态,该状态下原子在单位时间内发出的能量与吸收的能量相等。
因此,电磁能量密度ρ(T),即空腔每单位体积的能量是恒定的。该能量密度来自频率从0到无穷大的所有波。可知ρ(T)与绝对温度T的4次方成正比,即ρ(T) = αT4。故总能量和向外的辐射都会随着温度的升高而显著增加。因此,当空腔温度较高时,小孔看起来非常明亮,而在非常低的温度下,小孔则完全变黑。
不同的频率ν对能量密度的贡献ρν(ν, T)构成了所谓的光谱能量密度(spectral energy density)。经验表明,该密度在空腔中的各点都相同,且与空腔的几何形状、体积,以及构成空腔壁的材料无关。因此可将其视为关于ν和T的普适函数。要确定这一函数,只需在空腔壁上钻一个小孔,并结合ν和T,对测量到的辐射进行函数分析。
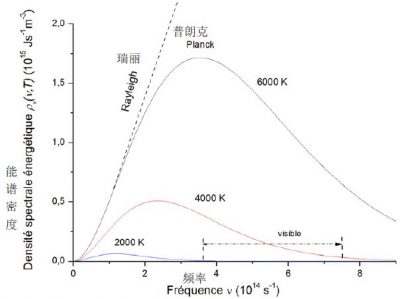
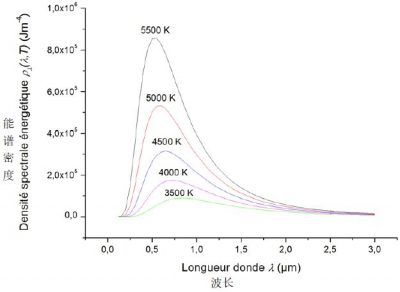
在特定温度下,观察到函数ρν(ν, T)随频率变化呈现钟形曲线(图2),在频率值νm = 5.879 ×1010T(s-1)处存在显著的最大值,该值随开氏温度K(Kelvins,开尔文)线性增加。这解释了辐射体的颜色变化:铁在680℃(953K)时呈暗红色,在810℃时呈浅红色,在1000℃时呈黄色,在1200℃时呈白色[1]。
3. 黑体辐射的能量
空腔内的能量密度难以直接测量,需通过测量腔体表面小孔S辐射的能量流来代替。要测量该通量,可在孔前放置一个传感器,传感器的加热速率能够反映其吸收辐射的能量。通过表面辐射的能量流定义为:在单位时间内和单位面积上穿过该表面的能量。如果所有的辐射都以速度c垂直于表面S逃逸(图3a),则体积成分Sc dt将在时间dt内穿过表面。因此,相应的能量为ρSc dt。通过除以时间和表面积,我们将获得与总能量密度ρ直接相关的能量流ρc。
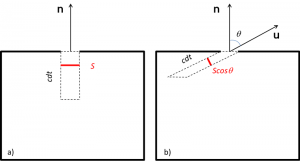
实际上,通过表面积S的能量受到一个因子¼的影响,该因子源于如下的几何学问题:在空腔内部,辐射经过多次反射,会以各向同性的方式分布在所有方向上。因此,实际上只有一小部分辐射在单位矢量u表示的方向附近以与法线n成角度θ的方式出射(图3b)。更准确地说,在u周围的非常小的立体角[2]中,只有总辐射的dΩ/4π可以介入。此外,同上文案例一样,必须考虑图3b中倾斜圆柱体的体积成分在时间dt内穿过表面的量。该圆柱体在传播方向Scosθ处存在法向截面和法向长度c dt。故可得体积S cosθ cdt。因此,单位时间内在单位面积上输出的基本流量为dΦ=ρ=ρc cosθ dΩ/4π。在洞外的半空间上,所有辐射方向cosθ/4π的总和,即等于¼,根据1871年实证发现的斯特藩-玻尔兹曼定律(Stefan-Boltzmann’s law),总能量通量为:
Φ = ρ c/4 = σT4 (其中σ = 5.670×10-8 W.m-2.K-4)
其中,频率为ν的辐射对该通量的贡献等于ρν(ν, T)c/4,并且可以测量。需要注意的是,黑体发出的辐射完全独立于由小孔射入、并被完全吸收的入射辐射。1884年,斯特藩(Stefan)的博士生路德维希·玻尔兹曼(Ludwig Boltzmann)为这种辐射奠定了热力学理论基础,但直到1901年,马克斯·普朗克(Max Planck)才利用量子理论阐明了这一基础(见《焦点》)。
4. 发射和吸收,基尔霍夫定律
根据定义,某表面如果吸收了某个频率域中的全部电磁辐射,则会在该频率域中呈现黑色。然而,该表面能够通过热辐射,重新向外发射电磁波。温度为T时,表面积S辐射的通量Φ等于相同温度下空腔壁上表面积相同的小孔发射的通量。如果小孔被黑色表面封闭(图3),则空腔必然呈现热平衡状态,从黑色表面到空腔内部的流量与黑色表面从空腔吸收的平均流量大小相等,方向相反。
类似地,如果温度为T的非黑体吸收了其入射辐射的一小部分a,则该物体在相同温度下发出的辐射通量将是黑体在该温度下发出的通量的一倍。该系数a即为吸收率(absorptivity)。发射率(emissivity),即在相同温度下,从一个表面发射的辐射与黑体发射的辐射之比,用系数e表示。基尔霍夫定律(Kirchhoff’s law)指出,这两个系数是相等的,即a = e。对于像镜子一样的完美反射器,a = 0,因此e = 0,即完美反射器不会出现热辐射。
通常来说,最好将黑体的光谱能量密度表示为波长λ = c/ν的函数,而不是波的频率ν的函数。波长函数ρλ(λ, T)很容易从频率函数ρν(ν, T)推导得来(详见《焦点》)。如图4所示,不同温度下能谱密度关于λ的函数图象呈现出钟形曲线。
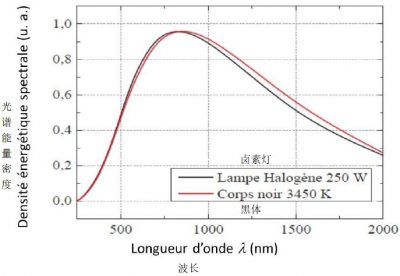
由图5可知,卤素灯在3450 K时的辐射与黑体(e = 1)在该温度下的辐射完全一致。
在常温下,材料会辐射红外线。材料的发射率不仅取决于波长,还取决于其表面状态。通常来说[3],对于抛光铝箔,e(λ= 3µm)= 0.09,e(λ = 10µm) = 0.04。如果观察各类材料对所有波长的总发射率e,可得到如下数据:抛光金的e = 0.02,沙子的e = 0.60,雪的e = 0.80,液态水的e = 0.96,白纸的e = 0.70-0.90,黑纸的e = 0.90,人类皮肤的e = 0.98。土壤和液态水的发射率接近1,有助于通过卫星红外成像确定地面温度。
5. 维恩定律及其在天体物理学中的应用
图4所示的ρλ(λ, T)曲线显示了由维恩定律(Wien’s law)得出的λm的最大值:
λmT = 0.201 hc/kB = 2.896×10-3 m.K
其中h是普朗克常量,c是真空中的光速,kB是玻尔兹曼常数(详见《压强、温度和热量》)。该表达式非常简单,常用于估测表面温度。
-太阳表面温度
太阳表面可以看作是黑色表面的一次近似;当λm = 0.501 μm时,太阳辐射的密度ρλ(λ, T)最大。根据维恩定律,可以推导出太阳表面的温度TS = 5777 K。
-太阳辐射到地球的热通量
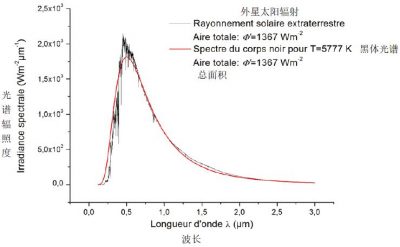
如将太阳表面近似为黑体表面,则太阳辐射的通量遵循斯特藩-玻尔兹曼定律:Φ0 =σT4。因此,半径R0 = 7×105 km的太阳发出的总功率P0等于该通量乘以半径为R0的球体表面积,即P0 = 4πR02Φ0。在距离太阳L处,相同的功率分布在半径为L的球体上,其局部通量Φ’ = P0/(4πL2)=Φ0(R0/L)2。已知地球与太阳的平均距离L = 1.5×108 km,可得到出通量Φ’= 1367 W/m2。图6显示了在大气层外测得的太阳实际发射光谱,将其与普朗克定律的理论表达式进行比较,发现该光谱与T = 5777 K时的 ρλ(λ, T) 几乎重合。与理论黑体曲线的几个最显著的偏差是由太阳表面的元素发射线和吸收线,主要是氢的巴尔默线[4]导致的。
-地球表面的辐射温度
关于该主题,文章《地球的气体外壳》已进行过讨论。
-来自宇宙的化石辐射
彭齐亚斯(Penzias)和威尔逊(Wilson)在1964年发现了类似于黑体的化石辐射(fossil radiation)的存在,其最大值λm =1.06 mm,对应温度为2.73 K。这种辐射具有非常低的各向异性,在不同方向上测得的辐射值变化非常小。该发现为大爆炸(big-bang)模型提供了重要支持,该模型假设宇宙随着时间的推移不断膨胀并冷却。根据这一理论,宇宙辐射自初级时期以来就一直存在。在初级时期,宇宙温度约为4000 K,由电子和质子组成。这种由电子和质子组成的等离子体与全频率的电磁辐射发生强烈的相互作用,因此物质和辐射处于平衡态。之后,宇宙冷却到3000 K,形成一种主要由氢原子组成的物质,黑体辐射作用仅在氢的特征频率下进行。因此,大部分电磁辐射与物质解耦,宇宙通过绝热膨胀冷却到2.73 K,该过程和气体膨胀相类似。在解耦后,物质演化成更重的原子,并自发组织形成星系、恒星和尘埃云,其发出的电磁辐射也成为宇宙黑体辐射的一部分。

图7显示了这种宇宙辐射随频率变化的通量谱,该通量谱于1989年由COBE卫星[5]测量得出。如图所示,在T = 2.726 K的实验条件下,由普朗克公式(见《焦点》)计算得出的能谱密度ρν(ν, T)理论曲线与卫星测量值完美重合。化石辐射存在于宇宙各处,目前总辐射量约为每立方厘米500光子。
6. 热成像摄像机的应用
热成像摄像机[6]捕捉发射体发出的红外线,这些红外线随温度的变化而有所不同。这证明了根据黑体辐射定律测温的可行性。其具体工作机制如下:可拆卸的过滤器可将辐射整合到光谱大气透明窗口中,该系统再将辐射功率转换成数字或模拟信号,计算机收集这些信号,将其转录为温度并生成图像,称为“热像图”。
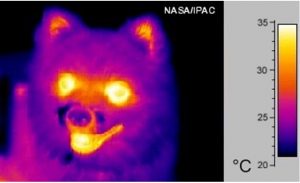
红外辐射的波长依赖于温度,在室温下(约300 K),根据维恩定律得出的最大辐射约为λ ~ 10 µm。因此,热成像相机的传感器测量的红外辐射,实际上是在λ = 10 µm附近选定一波长范围,并测量该范围内所有辐射的ρλ(λ, T)函数值之和得到的。相机产生的颜色是假颜色,将颜色与辐射强度相关联,便于直接读取温度。热成像图像中的每种颜色都有相应的温度,本文封面照片上的热成像和图8中小狗的热成像都是如此。
热成像摄像机的使用场景十分广泛:在救援人员难以到达的地区,可用其搜索地震受害者及其位置;消防员可用其检测即将出现或正在燃烟的火灾;公司可用其检测建筑隔热材料的薄弱点;机场可用其检测疑似发热病例;科研人员可用其研究蝙蝠等夜间活动的物种,从而避免使用可见光源干扰它们。
参考资料及说明
封面照片:英国地铁口的热成像[来源:branestawm2002,CC BY- Visual Hunt]
[1] http://www.thethermograpiclibrary.org/index.php?title=Table_emissivated_in_thermography
[2] 就像角度代表单位半径圆的弧长一样,立体角代表单位半径球面上的一部分曲面。对于在点O处的观察者来说,看到物体的立体角是物体的表观表面在以O为中心的单位半径球体上的投影。例如,在封闭的空腔内,看到空腔的立体角是单位半径球体的总表面积,即4π。
[3] http://www.thethermograpiclibrary.org/index.php?title=Table_emissivated_in_thermography
[4] 埃利·贝洛瑞斯基(Elie Belorizky),《量子力学导论》(Introduction to Quantum Mechanics),杜诺德(Dunod),巴黎(Paris),2003.
[5] https://en.wikipedia.org/wiki/Cosmic microwave background
[6] 不要将其与用于简单夜视的所谓红外相机混淆,红外相机工作的波长更短(1 m),不能用于热测量。
环境百科全书由环境和能源百科全书协会出版 (www.a3e.fr),该协会与格勒诺布尔阿尔卑斯大学和格勒诺布尔INP有合同关系,并由法国科学院赞助。
引用这篇文章: BELORIZKY Elie, PIQUE Jean-Paul (2024年4月23日), 黑体的热辐射, 环境百科全书,咨询于 2025年3月31日 [在线ISSN 2555-0950]网址: https://www.encyclopedie-environnement.org/zh/physique-zh/thermal-radiation-of-black-body/.
环境百科全书中的文章是根据知识共享BY-NC-SA许可条款提供的,该许可授权复制的条件是:引用来源,不作商业使用,共享相同的初始条件,并且在每次重复使用或分发时复制知识共享BY-NC-SA许可声明。